If z1 is a complex number other than -1 such that |z1| = 1 and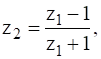 
then show that the real parts of z2 is zero.
Given:
⇒ 
Let us assume z1=x+iy
⇒ |Z1|=1
⇒ 
⇒ x2+y2=1-------------------(1)
⇒ 
⇒ 
⇒ 
⇒ 
⇒ 
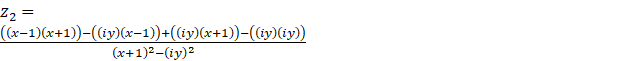
We know that i2=-1
⇒ 
⇒ 
⇒ 
⇒ 
⇒ 
∴ z2 is an imaginary one.
21