Solution of Chapter 8. Motion (NCERT Science Exemplar Book)
Chapter Exercises
Multiple Choice Questions
Short Answer Type
Long Answer Type
21
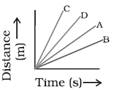
Using following data, draw time - displacement graph for a moving object:
Time (s) | 0 2 4 6 8 10 12 14 16 |
Displacement (m) | 2 4 4 4 6 4 2 0 |
Use this graph to find average velocity for first 4 s, for next 4 s and for last 6 s.