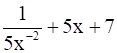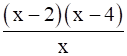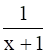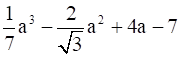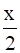Solution of Chapter 2. Polynomials (NCERT Mathematics Exemplar Book)
Chapter Exercises
Exercise 2.2
Exercise 2.4
8
If p(
20
If
24
Factorise:
2
24
Factorise:
24
Factorise:
24
Factorise:
3
30
If