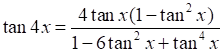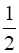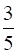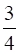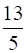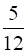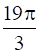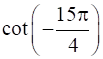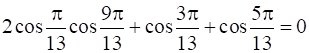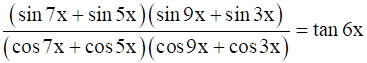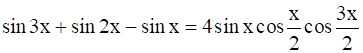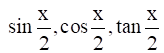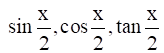Solution of Chapter 3. trigonometric functions (NCERT - Maths Book)
Chapter Exercises
Exercise 3.1
Exercise 3.2
Exercise 3.3
6
Prove 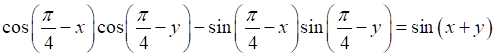
7
Prove 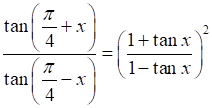
8
Prove 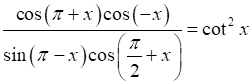
9
Prove 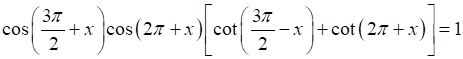
11
Prove 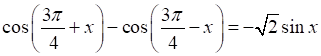
16
Prove that ![]()
17
Prove that ![]()
18
Prove that 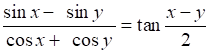
19
Prove that ![]()
20
Prove that ![]()
21
Prove that ![]()
23
Prove that